Difference between revisions of "Lensed cib"
(→Simulation Products: adding links to lensed CIB simulation products on CITA servers) |
|||
| (3 intermediate revisions by the same user not shown) | |||
| Line 3: | Line 3: | ||
= Simulation Products = | = Simulation Products = | ||
| − | Unlensed CIB mocks from this work can be accessed [http://mocks.cita.utoronto.ca/data/lensed_cib/unlensed here], and lensed CIB mocks can be accessed [http://mocks.cita.utoronto.ca/data/lensed_cib/lensed here]. | + | Unlensed CIB mocks from this work can be accessed [http://mocks.cita.utoronto.ca/data/lensed_cib/unlensed here], and lensed CIB mocks can be accessed [http://mocks.cita.utoronto.ca/data/lensed_cib/lensed here]. Python scripts for post processing are available [http://mocks.cita.utoronto.ca/data/lensed_cib/post_processing_scripts here]. |
<!-- Note that the Lensed and unlensed CIB simulation products can be found on CITA servers at /fs/lustre/project/act/mocks/lensed_cib/ --> | <!-- Note that the Lensed and unlensed CIB simulation products can be found on CITA servers at /fs/lustre/project/act/mocks/lensed_cib/ --> | ||
= Supplementary Figures = | = Supplementary Figures = | ||
| − | ... | + | <gallery mode="packed-hover" heights="500px"> |
| + | File:slice_lensing_vFeb2024.png | [[Media:slice_lensing_vFeb2024.png|Slice Lensing]] | ||
| + | </gallery> | ||
| + | <b>Figure 1:</b> CIB intensity, the corresponding lensing potential kernels, as well as the RMS deflection for each redshift shell (<math>\Delta z = 0.2</math>) within our Websky CIB model. The CIB intensity increases from <math>z = 0</math> to <math>z = 1.4</math>, peaks around <math>z = 1.4</math> to <math>1.6</math>, then decreases until it is almost non-existent by <math>z = 4</math>. The lensing kernels, defined as <math>W_{z_i^{\text{src}}}^{\kappa} = \frac{3}{2} \Omega_m H_0 ^2 \frac{1+z}{H(z)}\chi(z) \big[ \frac{\chi(z_i^{\text{src}})-\chi(z)}{\chi(z_i^{\text{src}})}\big]</math> with <math>z_i^{\text{src}}</math> being the midpoint of each redshift shell in <math>z</math>-space, typically peak around half of its extent, although they display some skew towards where the CIB intensity is highest, especially as we integrate over more redshifts. The RMS deflection steeply increases at first from <math>0.34~\mathrm{arcmin}</math> for the first lensed shell, up to <math>1.7~\mathrm{arcmin}</math> for the last shell. | ||
| + | |||
| + | <gallery mode="packed-hover" heights="500px"> | ||
| + | File:deck_of_cards_v5.png | [[Media:deck_of_cards_v5.png|Deck of cards]] | ||
| + | </gallery> | ||
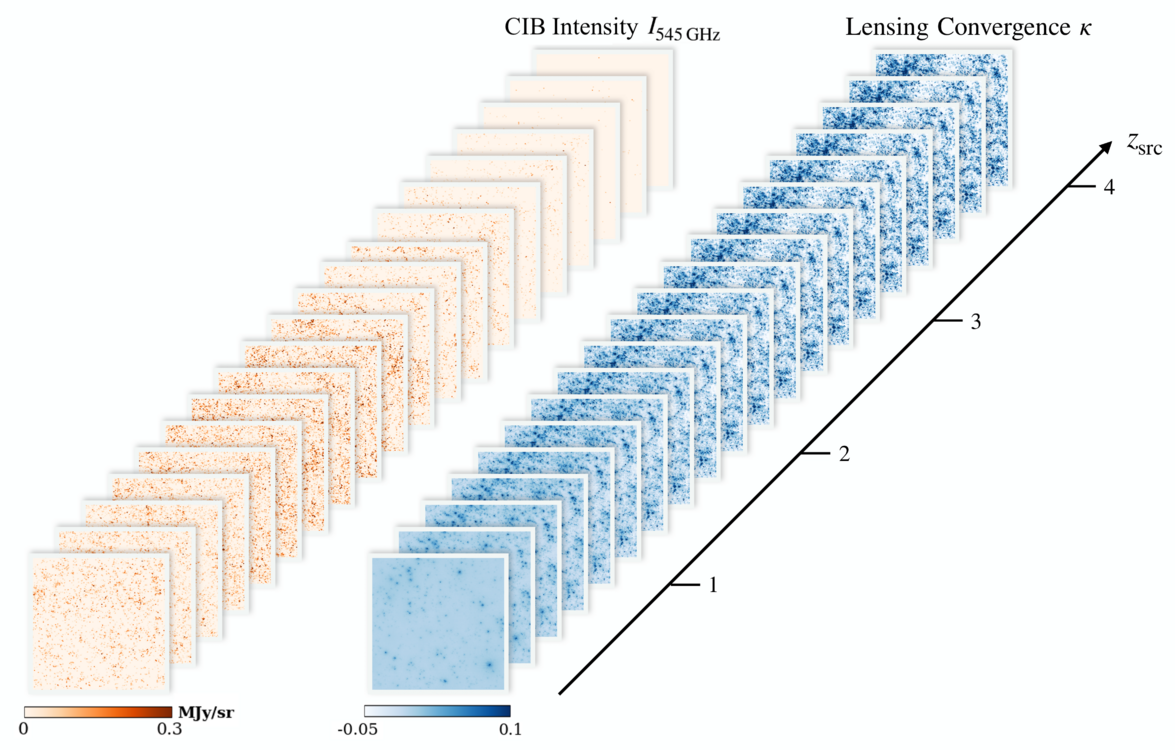
| + | <b>Figure 2:</b> A patch of unlensed CIB and its corresponding lensing convergence for each redshift shell <math>\Delta z = 0.2</math>. In our simulations, each unlensed CIB shell is lensed by a convergence shell to create lensed CIB shells, which are then summed up to produce the total lensed CIB map. This method mitigates the "self-lensing" effect substantially. Note that the CIB intensity visibly thins out by <math>z = 3</math>, while the integrated lensing convergence becomes brighter at higher redshifts. | ||
| + | |||
| + | <gallery mode="packed-hover" heights="500px"> | ||
| + | File:animation.gif | [[Media:animation.gif|Animation]] | ||
| + | </gallery> | ||
| + | <b>Figure 3:</b> HealPix maps of unlensed, deflected (no magnification), and lensed CIB for a small (<math>0.5^\circ \times 0.5^\circ</math>) patch of sky centered on <math>z = 1.1</math>. Here, a "lensed" galaxy has both been deflected and has had its flux density magnified appropriately. The arrows denote the direction and magnitude of deflection. The light circled patch (Unlensed and Deflected) and the dark circled patch (Deflected and Lensed) are the same small patch of sky emphasized. One can clearly see the deflection by comparing the Unlensed and Deflected, and the magnification effect by comparing the Deflected and Lensed. | ||
| + | |||
| + | <gallery mode="packed-hover" heights="500px"> | ||
| + | File:Websky_vs_Planck_unlensed_fluxcut_mean_no_err_Feb2024.png | [[Media:Websky_vs_Planck_unlensed_fluxcut_mean_no_err_Feb2024.png|WebSky vs Planck unlensed]] | ||
| + | </gallery> | ||
| + | <b>Figure 4:</b> Statistics of the unlensed CIB maps from the Websky simulations at the three <i>Planck</i> frequencies; top (blue) is 545 GHz, middle (green) is 353 GHz, and bottom (red) is 217 GHz. As we go to higher-order statistics, the Poisson regime becomes more evident as the spectra flatten out at <math>\ell > 1000</math>. We note that the Websky bispectra are mostly within <i>Planck</i> error bars even though only the power spectra were fit to match those of <i>Planck</i>'s. While we do not plot the error bars for Websky values as there is only one realization, one can estimate the level of uncertainty from the scatter especially for the bispectra and kurtosis. | ||
| + | |||
| + | <gallery mode="packed-hover" heights="500px"> | ||
| + | File:unlensed_vs_no_kapmax_with_gamma_smoothed_all_freq_fluxcut_Planck_mean_Feb2024.png | [[Media:unlensed_vs_no_kapmax_with_gamma_smoothed_all_freq_fluxcut_Planck_mean_Feb2024.png|WebSky unlensed vs no kapmax with sigma smoothed]] | ||
| + | </gallery> | ||
| + | <b>Figure 5:</b> The effect of gravitational lensing on CIB statistics using lensing convergence maps smoothed at the pixel level. While the power spectra are changed by less than 2% for all three frequencies, the bispectra and kurtosis change by 10 to 40% at low <math>\ell</math>. The apparent discrepancy between frequencies for the bispectra and kurtosis arises due to the relatively high flux cut values for <i>Planck</i> at lower frequencies. | ||
| + | |||
| + | <gallery mode="packed-hover" heights="500px"> | ||
| + | File:Websky_vs_Planck_with_gamma_lensed_bl_all_ebars_Feb2024.png | [[Media:Websky_vs_Planck_with_gamma_lensed_bl_all_ebars_Feb2024.png|Websky vs Planck with gamma lensed]] | ||
| + | </gallery> | ||
| + | <b>Figure 6:</b> Comparison of unlensed and lensed Websky bispectra with <i>Planck</i> measurements. Points are computed at the same set of central <math>\ell</math> values but are horizontally offset for clarity. The lensed values are slightly larger than unlensed values and hence closer to <i>Planck</i> values. | ||
Latest revision as of 15:48, 15 August 2024
On this page you will find simulation products and supplementary figures to accompany the paper Exploring the Non-Gaussianity of the Cosmic Infrared Background and Its Weak Gravitational Lensing - J. Lee, J. R. Bond, P. Motloch, A. van Engelen, G. Stein (2024).
Simulation Products
Unlensed CIB mocks from this work can be accessed here, and lensed CIB mocks can be accessed here. Python scripts for post processing are available here.
Supplementary Figures
Figure 1: CIB intensity, the corresponding lensing potential kernels, as well as the RMS deflection for each redshift shell ([math]\displaystyle{ \Delta z = 0.2 }[/math]) within our Websky CIB model. The CIB intensity increases from [math]\displaystyle{ z = 0 }[/math] to [math]\displaystyle{ z = 1.4 }[/math], peaks around [math]\displaystyle{ z = 1.4 }[/math] to [math]\displaystyle{ 1.6 }[/math], then decreases until it is almost non-existent by [math]\displaystyle{ z = 4 }[/math]. The lensing kernels, defined as [math]\displaystyle{ W_{z_i^{\text{src}}}^{\kappa} = \frac{3}{2} \Omega_m H_0 ^2 \frac{1+z}{H(z)}\chi(z) \big[ \frac{\chi(z_i^{\text{src}})-\chi(z)}{\chi(z_i^{\text{src}})}\big] }[/math] with [math]\displaystyle{ z_i^{\text{src}} }[/math] being the midpoint of each redshift shell in [math]\displaystyle{ z }[/math]-space, typically peak around half of its extent, although they display some skew towards where the CIB intensity is highest, especially as we integrate over more redshifts. The RMS deflection steeply increases at first from [math]\displaystyle{ 0.34~\mathrm{arcmin} }[/math] for the first lensed shell, up to [math]\displaystyle{ 1.7~\mathrm{arcmin} }[/math] for the last shell.
Figure 2: A patch of unlensed CIB and its corresponding lensing convergence for each redshift shell [math]\displaystyle{ \Delta z = 0.2 }[/math]. In our simulations, each unlensed CIB shell is lensed by a convergence shell to create lensed CIB shells, which are then summed up to produce the total lensed CIB map. This method mitigates the "self-lensing" effect substantially. Note that the CIB intensity visibly thins out by [math]\displaystyle{ z = 3 }[/math], while the integrated lensing convergence becomes brighter at higher redshifts.
Figure 3: HealPix maps of unlensed, deflected (no magnification), and lensed CIB for a small ([math]\displaystyle{ 0.5^\circ \times 0.5^\circ }[/math]) patch of sky centered on [math]\displaystyle{ z = 1.1 }[/math]. Here, a "lensed" galaxy has both been deflected and has had its flux density magnified appropriately. The arrows denote the direction and magnitude of deflection. The light circled patch (Unlensed and Deflected) and the dark circled patch (Deflected and Lensed) are the same small patch of sky emphasized. One can clearly see the deflection by comparing the Unlensed and Deflected, and the magnification effect by comparing the Deflected and Lensed.
Figure 4: Statistics of the unlensed CIB maps from the Websky simulations at the three Planck frequencies; top (blue) is 545 GHz, middle (green) is 353 GHz, and bottom (red) is 217 GHz. As we go to higher-order statistics, the Poisson regime becomes more evident as the spectra flatten out at [math]\displaystyle{ \ell \gt 1000 }[/math]. We note that the Websky bispectra are mostly within Planck error bars even though only the power spectra were fit to match those of Planck's. While we do not plot the error bars for Websky values as there is only one realization, one can estimate the level of uncertainty from the scatter especially for the bispectra and kurtosis.
Figure 5: The effect of gravitational lensing on CIB statistics using lensing convergence maps smoothed at the pixel level. While the power spectra are changed by less than 2% for all three frequencies, the bispectra and kurtosis change by 10 to 40% at low [math]\displaystyle{ \ell }[/math]. The apparent discrepancy between frequencies for the bispectra and kurtosis arises due to the relatively high flux cut values for Planck at lower frequencies.
Figure 6: Comparison of unlensed and lensed Websky bispectra with Planck measurements. Points are computed at the same set of central [math]\displaystyle{ \ell }[/math] values but are horizontally offset for clarity. The lensed values are slightly larger than unlensed values and hence closer to Planck values.